Predicting Arrow Penetration on Real Animals: A First Test of the Tissue Penetration Index (TPI)
Is there a way to predict, not infallibly, but with a high degree of reliability, the ability of a particular bow/arrow/broadhead combination to penetrate real animal tissue (hide, meat, fat, connective tissue, etc., and bone) under real hunting conditions? The answer to this question becomes of particular importance when truly “big” game is hunted with the bow and arrow.
Being able to predict such performance would also be of help in determining what combinations will assure “adequate performance” on “normal” size animals when the shot placement is less than ideal. Exceptional arrow penetration then becomes necessary to achieve a quick, clean, killing shot. Such information would also be of more than passing interest to game department officials and lawmakers when considering the “humanity” of bowhunting and for developing realistic bowhunting regulations.
I have been seeking an answer to this one question of “arrow and broadhead effectiveness” for years. I would like to tell you that I have found the “ultimate” answer but, alas, I’m not even sure that there is an “ultimate” answer. There is little question that the most important factors determining broadhead “lethality” are shot placement, broadhead sharpness and adequate penetration. Regardless of one’s skill, shot placement can never be guaranteed. Animal reflexes are simply faster than the very fastest of arrows, and occasionally most bowhunters “muff” even an easy shot.
Sharpness is easy enough to achieve, with a little effort, but, all else being equal, adequate (surplus) penetration is the factor that can often render a marginal or poor hit quickly effective. As the size of the animal being hunted increases, the importance of adequate penetration is magnified. This being the case, how do we go about assuring that the equipment we use will deliver the amount of penetration needed when hunting truly large animals or “when everything goes wrong”?
There are some factors which decidedly affect the potential for adequate penetration, and these factors can be collated into a series of equations which appear to offer a reasonable predictor of penetration when comparing one arrow/broadhead combination to another.
Following the research on broadhead performance, conducted under the auspices of the Natal Parks Board in South Africa in 1984 and 1985, which I was fortunate to participate in, I became almost consumed by a desire to understand, as fully as possible, the factors which affect broadhead and arrow performance on real animal tissue. It is obvious that a number of factors are at play in determining the penetration capability of an arrow/broadhead combination on real tissue.
Using the data from that original study, the largest ever conducted on real animals, I have struggled to analyze it from all possible viewpoints. From that information, and data collected since then, I have gradually formulated a theory. This theory is based on the detailed examination of the wound channel of several hundred shots on real game animals, not on the testing of a few arrows on substances as “real” as styrofoam, ethafoam, cardboard, wood, ballistic gel, de-boned beef sides, etc.
Some have used such test on artificial medium to try and advance a particular concept as the “ultimate answer” to predicting penetration. I will, however, continue to treat my concept, which I have named the “Tissue Penetration Index”, or “TPI”, as a theory, not the final answer to end all. Much field research, and possibly some lab studies, remain to be done at this point. I do, however, feel that use of the TPI comes a great deal closer to correlating with actual measured performance on real animals than any other “yardstick” I know of.
The purpose of this article is to distribute the results of my work to date on the TPI, and the results of the first field test of the TPI, so that others may examine it and, hopefully, refine it through further analysis of real shots on real animals. Such research is vital for the sport.
Each year an increasing number of bowhunters are going afield after the larger species of game. Without a thorough understanding of what is required to achieve sufficient penetration, on large, difficult to penetrate, and potentially dangerous animals, there will be a great deal of needless wounding of game. This will surely result in a “bad publicity rap” for bowhunting. Several such cases have already occurred here in Africa. Should it continue, this may result in unreasonable restrictions on bowhunting, or total bans on bowhunting in some areas.
Even on slightly built animals, understanding how to optimize arrow performance will result in an increase in arrow lethality. This “extra performance margin” becomes particularly important when Mr. Murphy throws his “law” into motion against us, and the arrow does not hit where we intended, ie: s–t happens, folks!
The TPI is an attempt to analyze the principal factors affecting arrow penetration, in terms of the laws of physics, by use of mathematical formulas. The TPI couples the arrow momentum with the calculated mechanical advantage of the broadhead (M.A.) and a “drag factor” based on shaft diameters less than, equal to, or greater than the broadhead ferrule diameter.
By definition from physics, momentum, and not kinetic energy, is the correct formula to measure the directional (in this case, forward) “impulse” of a body in motion. It is the force exerted over a period of time in one specific direction, ie: a unidirectional force vector.
Kinetic energy (K.E.) is scalar, or nondirectional, in nature, and includes all the types of energy of a body in motion. K.E. has no direct bearing on penetration. A tuning fork, once struck, has high kinetic energy (it can shatter a crystal wine glass), but has almost no momentum. It would make a darn poor penetrator of tissue!
For those with a scientific bend, the formula for the momentum of an arrow is: arrow weight in pounds times arrow speed in feet per second divided by the acceleration of gravity. In equation form, it would be represented as:
Momentum = (arrow weight on grains/7000) X arrow speed in fps ÷ by 32 feet per second per second
The second factor in the TPI is the mechanical advantage (M.A.) of the broadhead. A single blade broadhead, with a straight taper, can be viewed, in terms of physics, as a “simple machine”. It is a series of inclined planes. How the ferrule tapers into the blade is a factor, but can be ignored in comparing one broadhead to another if the two ferrules are relatively the same degree of taper. It is important that the broadhead is of quality construction. If any portion of a head bends of breaks on impact with any tissues (including bone), it negates any methodology of predicting penetration and almost assures poor performance.
For a simple broadhead design, the approximate M.A. may be calculated by dividing the length of the cutting blade (from tip to widest point of the edge) by one half the cutting width of the broadhead (distance from the centerline of the ferrule to the widest point on the cutting edge) and multiplying by the number of cutting blades. In equation form this would be represented as:
M. A. = Length of cutting blade to widest point
1/2 width of blade cut X number of cutting blades
The M.A. of single blade heads and some multi-blade heads is easy to calculate. Broadheads of complex construction, with blades of various plane angles, “bone breaker” tips, etc. are difficult to calculate. Perhaps actual penetration test in a uniform medium, such as ballistic gel, using a projectile of uniform mass and impact velocity, would yield a “relative resistance factor”. This “relative factor” could be used in place of the calculated M.A. to ascertain the TPI. Testing of this degree is certainly beyond my financial means. However, I feel this concept is plausible and warrants investigation.
The third factor used to arrive at the TPI is a “shaft drag factor”. This factor was derived from the data accumulated during the Natal broadhead tests. It was determined by comparing the average penetration depth when arrows of equal momentum, all using the same broadhead, were shot on shafts with diameters smaller than the broadhead ferrule diameter, equal to the ferrule diameter and greater than the ferrule diameter. The “multiplication factors” thus arrived at are 1.0, .9, and .6 respectively. Obviously “shaft drag” is a major factor in penetration, equally as important as arrow momentum and quality and M.A. of the broadhead.
The TPI is merely a relative numerical value arrived at by multiplying the momentum of the arrow by the M.A. of the broadhead and multiplying the product by the “shaft drag factor”. The TPI formula appears to work as a predictor of penetration on real tissue, however much testing remains to be done to establish the level of TPI needed for various species of animals. Initial tests indicate that the TPI does accurately predict the penetration capability (in real tissue) of various arrow/broadhead combinations relative to each other.
I recently returned from Mozambique, where I was able to test some arrows on fresh (immediately after the kill) cape buffalo carcass. All testing was done with the 190 grain Grizzly broadhead (which I believe is the very best “Big Game” broadhead available, based on the Natal study) and a modified version of the Grizzly with a narrower cut radius (giving a higher mechanical advantage). This eliminated differing broadhead quality and structure as a variable.
Working with arrows of differing mass, velocity, and shaft diameters, I calculated the K.E., momentum, momentum times the M.A., and the TPI for each combination prior to testing the penetration. A heavy, thin, shaft (900 grains) and broadhead of high M.A. was able to penetrate the ribs and plunge through the opposite (offside) ribs of the buffalo carcass. This combination had the highest “scores” in all categories; K.E., M.A., and TPI.
The real “test” of the TPI came when evaluating the other combinations. There, some of the combinations with lower K.E.’s and momentum consistently “out penetrated” those with higher K.E.’s and momentum levels by a substantial margin. This performance was accurately predicted by the TPI. On even the massive Cape Buffalo, some of these combinations will give reliable penetration, through the ribs and deep into the thorax, and would be lethal on a perfect broadside chest shot.
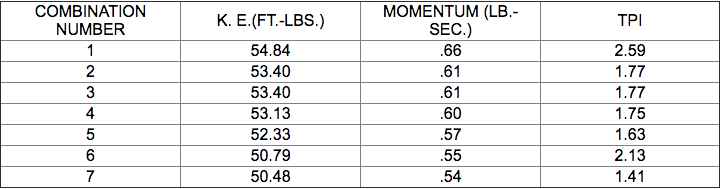
Enclosed are graphs comparing the K.E., Momentum, Momentum times M.A., and the TPI to the actual measured penetration on the buffalo with seven arrow mass/velocity/M.A./drag factor combinations. Examination of these reveals a poor correlation for both K.E. and momentum as a predictor of penetration. There is some improvement when the broadhead’s M.A. is incorporated with the momentum (ie: the TPI without the shaft drag factor). The TPI offers a very high correlation as a predictor of tissue penetration.
The cape buffalo is nearly the perfect test animal for developing this performance data. The Cape Buffalo has ribs which overlap each other, and all broadside shots (as all these shots were) must penetrate a rib on entrance. The following table gives the K.E., momentum, and TPI for each of the combinations used in the testing.
Combinations number 2 and 3 are identical and serve as a control. Combinations number 6 and 7 are lighter arrows (654 and 650 grains) at the same velocity (187 fps), with modified Grizzly broadheads of the same (very high) M.A., but with number 6 having a shaft of diameter equal to the ferrule and number 7 with a diameter larger than the ferrule diameter.
This concept is rather complex, and a great deal of work needs to be done to develop information on the TPI needed to assure adequate penetration on various species of game, particularly on adverse angle hits (which can and do happen).
Using the TPI formula, a 60-pound longbow firing a 788 grain compressed cedar arrow, with a 190 grain Grizzly broadhead, at 148 fps has only 38.34 ft. lbs of K.E., .52 lb.-sec. of momentum, but has a TPI of 1.50. That combination was used to repeatedly shoot through the scapula of a large zebra stallion and through the thorax to the off side, often breaking off-side ribs (never failing to penetrate the scapula and completely through the thorax). This was compared to a compound firing a 555 grain aluminum shafted Black Diamond at 229+ fps. This combination gives 65.21 ft. lbs. of K.E., a momentum of .57 lb.-sec., but a TPI of only 1.27. That compound was, at best, able to penetrate only 5 to 8 inched beyond the scapula, and occasionally failed to penetrate the scapula at all on that same zebra carcass.
The 60# longbow/788 grain arrow/Grizzly broadhead was also compared to a high energy compound firing a 450 grain carbon arrow tipped with a three blade head, with a cut width of 1 1/8″ and a cutting blade length of 2″, at a velocity of 259+ fps. This combination yields 76.56 ft. lbs. of K.E., .52 lb.-sec. of momentum, but a TPI of only 0.62. It was unable to penetrate the zebra scapula.
Penetration of this combination (compound, lightweight carbon shaft, and 3 blade head) on all the larger antelope species is marginal at best. This bears out the reduced penetration capability of this set-up compared to the lower velocity longbow with its heavy arrow and single blade broadhead of high mechanical advantage. This even though the momentum of both combinations is identical (.52 lb.-sec.), and the compound has 76% more kinetic energy (67.56 ft. lbs. vs 38.34 ft. lbs.).
What we do know from the broadhead research in Natal is that multiblade heads are a poor choice for use on larger animals, even in the antelope class (because of the poor M.A.). We know that the quality and the design (mechanical advantage) of the broadhead is an important factor. We know that arrow mass is more important than arrow velocity (because arrow velocity is rapidly lost down range, but arrow mass remains constant through the arrow’s flight – helping to maintain the arrow’s momentum). We also know that bow draw weight DOES NOT translate directly to arrow velocity, penetration capability, or killing capacity of the arrow/broadhead combination. Some bows are simply more efficient than others. Any chronograph will prove that a properly set up compound bow will generate higher momentum levels with any given arrow mass than a conventional bow of equal draw weight.
I hope the reader will find this data of interest and of some use. As far as bowhunting legislation is concerned, I feel that placing unreasonable bow weight requirements or requiring the use of specific arrow shaft materials which must (because of their very nature) result in the use of lighter weight arrows would be detrimental to bowhunting.
These tests are further evidence that legislated requirements for a specific bow draw weight for specific size animals is a total fallacy, nor is legislation of a specific level of kinetic energy or arrow momentum the answer. They simply do not correlate with penetration in real tissue.
The TPI is still in a fledgling state, and sufficient testing has not been conducted to even consider its use as a “standard” of adequacy for the various animals, but it can be used as a guideline in calculating what should work reliably. At this stage, it would be better to require reasonable bow weight (and we do have much historical data to rely on here), arrows of significant mass, and restrict the broadheads used to those of excellent quality and high mechanical advantage (on the larger animals) as the “benchmark” for adequacy.
No one would argue that light arrows and multiblade broadheads are not adequate on the smaller animals – a great many are routinely taken with them. (Although I personally feel that use of “excess penetration capability” turns many a non-lethal hit into a very lethal hit, even when hunting light game.) The real issue is solely with the larger, heavier, and particularly the potentially dangerous species of game.
I also have reservations about the wide-spread requirement for minimum broadhead widths, particularly on the larger animals. The wider the broadhead, for a given length, the lower the M.A. of the head. With the larger animals, penetration is the problem. What is needed is a long, deep, wound channel rather than a wider, less deep, wound channel.
A blunt or round-nosed “solid” (non-expanding) rifle bullet, even of small caliber, driven at a moderate velocity, will kill the largest of animals when driven through the chest cavity. Even elephants, like Bell, Stiggand, Blunt, and others proved. (And, according to Peter Capstick, at least two elephants have been killed with the lowly .22 Long Rifle cartridge on heart shots!). Remember also that even the .600 Nitro Express’ solid bullet is less than 3/4″ in diameter. No one would question its ability to kill any animal on a chest shot!
I have used, and seen used, a large number of such bullets on animals, and have examined hundreds of such wound channels. Tissue damage and “tissue shock” are minimal from such a solid bullet’s wound when velocity is moderate. The animals so hit (where no bone or nerve center is hit) die from blood loss and/or lung collapse, just as with an arrow. Such a wound produces a rate of blood loss much slower than with a broadhead of similar width.
The American Indians frequently made very tiny flint broadheads. For many years, these were believed to be for use on small animals and, indeed, were commonly called “bird heads”. More recent archeological research has shown that these tiny broadheads were really used to kill the American Bison! Bison are substantial animals, with bulls often weighing in excess of 3000 pounds. With the weak bows used by the “Plains Indians” (those tribes cohabitating the great American prairie with the Bison), the only way to achieve adequate penetration was to reduce the force needed to penetrate to a minimum.
Just as the “Plains Indians” discovered long ago, with a broadhead tipped arrow, penetration is the problem on the larger species. They found that a wide broadhead, cutting a wide path, was useless, as it often failed to penetrate sufficiently to reach the vital areas. A small broadhead, penetrating deep into the thorax was sufficient to be quickly fatal.
A 3/4″ wide broadhead through the lungs will most assuredly kill more quickly than a similarly placed “solid” rifle bullet at moderate velocity. In the 1960s, before their use was legislated out of existence in the States, I used many very narrow broadheads, some as narrow as 1/2″, and have never once had one fail to kill any large animal hit. Perhaps this concept should also be “re-visited” and some valid research performed. Smaller broadheads have received a lot of “regulation” and “bad press” without any objective data to back up those restrictions.
I have devoted a great deal of time and effort into this research over the last twelve years, and feel that I most likely have the largest amount of data on arrow and broadhead performance on real animal tissue of anyone. It is my deepest hope that other independent, objective, researchers will take some interest in this work and conduct their own testing to expand the bowhunting community’s understanding of just what it takes for a hunting arrow to be effective under real field conditions. Truly, my feelings will not be hurt if the results are different from my data. It will all expand our knowledge of how to be better bowhunters.
Copyright 1996 Dr. W.E (Ed) Ashby